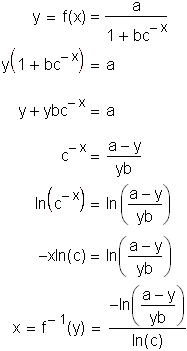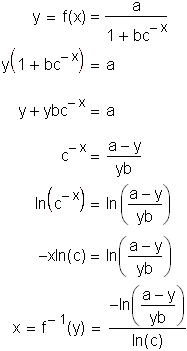Inverse Logistic Functions
If y = f(x) = a / (1 + b c –x) , then we solve for x in terms of y using the laws of logarithms, as follows:
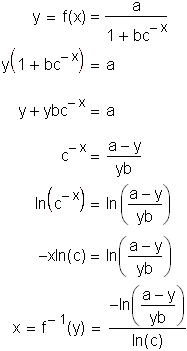
In typical applications of logistic functions, all three parameters a , b , and c are positive. The output y of the forward function f varies between 0 and the "carrying capacity" a :

Thus a – y , yb , and c are all positive for 0 < y < a . As a result, the two logarithms in the inverse function will have positive inputs, and the inverse will be defined for all y values in this range.