| Click here to download this file as a Word document. | |
| Click here to download this file as a PDF document. |
Mathematical Wunderkammern
William Mueller
1. Introduction: The Age of Wonder.
A giddy craze was sweeping across Europe
at the turn of the 17th century. The wealthy and the well-connected
were hoarding things—strange things—into obsessive personal collections.
Starfish, forked carrots, monkey teeth, alligator skins, phosphorescent
minerals, Indian canoes, and unicorn tails were acquired eagerly and
indiscriminately. Associations among these objects, if they were made at all,
often reflected a collector's personal vision of an underlying natural
"order". Critical taxonomy was rarely in evidence.
The enthusiasm that characterized such
collections was captured by Francis Bacon [1, p. 247], who ironically advised
"learned gentlemen" of the era to assemble within "a small
compass a model of the universal made private", building
|
... a goodly, huge cabinet, wherein
whatsoever the hand of man by exquisite art or engine has made rare in stuff,
form or motion; whatsoever singularity, chance, and the shuffle of things
hath produced; whatsoever Nature has wrought in things that want life and may
be kept; shall be sorted and included. |
These "cabinets" were proudly
displayed, and selected visitors were allowed to wander through the accumulated
"shuffle of things" to express their awe and admiration. Other
expressions, such as perplexity and incomprehension, were circumscribed by what
was, in pre-Enlightenment Europe, a well-practiced deference to presumptive
authority. Indeed, the cabinets can be seen as a sort of physical manifestation
of centuries-old religious and philosophical states of consciousness. These
first "museums" presented visitors with an opportunity to place the
era's flood of information within a comfortable psychological order. As the century
progressed, patrons would pay for the privilege of a carefully arranged
understanding of the world's troubling uncertainties.
|
|
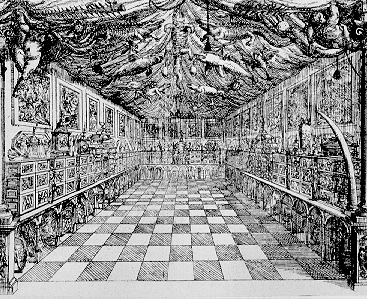
Figure 1. The museum of Manfredo Settala, Milan, ca. 1600.
Europe in 1600, one must remember, was
crossed by many unsettled intellectual currents. The Renaissance, which had
begun in Italy in the 14th century, had spread throughout Europe by the
end of the 16th, reviving the continent's slumbering passion for art
and learning. As the Renaissance progressed, however, it had transformed Europe
into a much more complicated place. By 1600, the consequences of so much
concentrated inquiry were beginning to show in the troubled creases across many
European brows. Explorers were regularly bringing back incredible, inscrutable
objects from the New World and the Far East; objects whose existence brought
into question the centrality of Europe and the primacy of its culture.
Simultaneously, the Scientific Revolution, led by the likes of Copernicus
(1473-1543), Bacon (1561-1626), and Galileo (1564-1642), was challenging the
centrality of the world itself. As the extraordinary scope of Geography and
Nature began to emerge, many traditional, comforting notions seemed to shrink
in comparison. It was a bit too much for some, and the profound unease stirred
up by such revolutionary changes gradually fueled a reaction. In extreme cases,
science was labeled Heresy. (Giordano Bruno was burned at the stake in 1600;
Galileo was imprisoned by the Inquisition and threatened with torture in 1633.)
Scientists, still in their early days of mixing powders and picking apart
anatomies, were not yet in a position to respond with assurance.
What is remarkable about this era is that,
even when every boundary seemed to be expanding beyond the known horizons, and
even when the objects from those far horizons suggested a world that was larger
than the imagination, there was nevertheless a desire to reach further. It was
daring, for what lay beyond was completely unknown. Commerce, politics, and
religion, each with its own agenda, tried to temper the excessive enthusiasm.
Nothing, however, could direct the collective fascination with the New World's
dark tumult. Curiosity cabinets proliferated across Europe. Eventually, these
cabinets became the first museums of science, natural history, medicine, and
art, depending on how curators chose to arrange their accumulated treasures.
What these first museums showed their patrons was that the New World—whatever
it was—was undoubtedly wonderful. The collections became known as wunderkammern,
or "wonder cabinets".
The emerging scientific community wasn't
entirely pleased with the craze for wunderkammern. Those who took the
Revolution seriously found the curiosity cabinets not only frivolous, but
reactionary—an impediment to the properly measured progress of scientific
reason. Wonder, they argued, ought to be lavished on the technical advances in navigation
that had allowed explorers to sail back and forth to the New World time and
again, not merely on the gewgaws that they had brought back. Galileo [15, p.
459] wrote with apparent distaste of the "curious little men" who
would gather
|
... a petrified crab, a desiccated
chameleon, a fly or spider in gelatin or amber, those small clay figurines,
supposedly found in ancient Egyptian burial chambers. ...Our poet errs as
much as would a painter who, purposing to depict a particular hunting scene,
were to clutter his canvas with conies, hares, foxes, goats, deer, wolves,
bear, lions, tigers, boars, hounds, greyhounds, leopards, and all manner of
wild beasts, clustering at will animals of the hunt with every sort of game,
such as to liken his painting more unto a representation of the entry into
the Ark of Noah than unto a natural hunting scene. |
Descartes [16, p. 20] gave the curiosity
cabinets an even more peremptory wave: "What we commonly call being astonished
is an excess of wonder that can never be otherwise than bad."
2. Mathematics in the Age of Wonder.
Mathematics, as might be expected, was an
eager participant in the excitement that marked the beginning of the 17th
century. By this time, a tremendously fruitful interchange had developed among
mathematics, science, engineering, and commerce. Practical problems that arose
in the burgeoning complexities of international trade, in navigation, in the
construction of cities, and in the logistics of warfare were leading to
fundamental new developments in algebra, geometry, and computation. As the
century progressed, mathematicians such as Harriot and Oughtred would
rediscover the algebraic techniques that Arab mathematicians had employed
during Europe's Dark Ages, rewriting them in a new language of symbols (much to
the chagrin of typesetters); Desargues, Pascal, and Descartes would completely
re-invent the way in which geometric problems were investigated; Napier would
introduce an efficient new tool for computation called the logarithm; and
Fermat would apply infinitesimal methods to the problem of finding the maximum
and minimum values of a function. By the century's end, all of these
developments would coalesce into one of mathematics' most lasting contributions
to practical science: The Calculus.
These contributions were widely noticed
and appreciated by the 17th century public. Indeed,
"mathematical" apparatus such as calculating machines, drawing
instruments, astrolabes, sundials, and the recently invented sector and slide
rule found their way into many of the cabinets of curiosity. Unnoticed by much
of the public, however, was mathematics' slow turn inward. This was, after all,
the "early modern" period of bold new experiments in abstraction.
Mathematical advances gradually became much less generally understood, even by
those who considered themselves to be active participants in the era's
intellectual life. The logic and systematic discipline within mathematics, as
well as the many practical applications that it produced, continued to impress,
but outsiders could no longer hope to share in the excitement of fundamental
new treatises the way they might have only half a century before. As a result,
there was not a rush to learn logarithms the way that there was a rush to taste
new teas from China.
Many mathematicians and scientists of the
Revolution found this to be an entirely appropriate state of affairs.
Mathematics and Science, as they were being newly imagined, were disciplined
endeavors proceeding along ineluctable paths of reason. Mathematics, in
particular, was not advanced through the wondrous contemplation of numerical or
geometrical gewgaws. Descartes [4, p. 47] saw mathematics as an exercise in
intellectual discipline, necessary for discovering unimpassioned truth. He
wrote:
|
As for myself, who am conscious of my
feebleness, I have resolved to observe constantly, in the search after
knowledge, such an order that, commencing always with the most simple and
easy things, I never take a step forward in order to pass the others, until I
believe that nothing more remains to be desired concerning the first. |
Descartes was removing himself as far as
he could from the unrestrained enthusiasms that characterize wonder, and his
sentiments were typical among mathematicians of the time. This view of
mathematics, as a strictly logical construct with little tolerance for
wanderers, would persist for centuries to come.
Descartes' resolve "to observe
constantly ...an order" must be contrasted with the obsessive
"observations" of the wunderkammern enthusiasts. Pascal [4, p.
58], who like Descartes cultivated more pious enthusiasms, remarks that:
|
...it is rare that mathematicians are
observant, and that observing minds are mathematicians. ...Mathematicians who
are mere mathematicians, have thus their understanding correct, provided
always that everything be well explained to them by definition and principle:
otherwise they are false and insupportable; for they are correct only on principles.
And minds of observation, if only observant, are incapable of the patience to
descend to the first principles of matters of speculation and imagination, of
which they have no experience... |
In other words, mathematicians, when doing
mathematics properly, keep their heads down and their vision focused: A
conception of mathematics as a terribly sober business.
It was, however, a conception that was
undeniably productive. The historian Morris Kline [27, p. 391] has written:
|
By the end of the [17th]
century, mathematics had undergone such extensive and radical changes that no
one could fail to recognize the arrival of a new era. The European
mathematicians produced far more between about 1550 and 1700 than the Greeks
had done in roughly ten centuries. |
Many mathematicians were eager to see
these accomplishments included among the "wonders" of the day.
Pascal, for example, did not object to having his adding machine appear among
the crocodile skins of many wunderkammern.
Most of the objects on the mathematical
horizon in 1600, however, had been in sight for some time: algebraic equations,
rows of calculations, trigonometric constructions, smoothly varying plane
curves. Mapping the mist-covered routes between these objects, and negotiating
the rocky shores that appeared when the mists began to clear, surely made for
many satisfying mathematical voyages. The existence of the routes themselves,
however, could not have been in serious doubt, and the voyages must not have
seemed especially risky. Much of the time in mathematics, it was if western
routes to the Indies were being found as expected.
It is thus difficult to describe the
mathematics of the early 17th century in quite the same terms as
those used to describe the revolutionary intellectual discoveries shaping the
larger culture. The new era in mathematics that would come to pass by the end
of the century was the result of a long, deliberate surveying of the
mathematical landscape. There was no Magellan, Drake, or Hudson to bump into
calculus; no Bacon, Hobbes, or Locke to repudiate the entire inherited
intellectual system; no Copernicus, Galileo, or Kepler to re-imagine the
mathematical universe. The mathematical discoveries of the early 17th
century, though profound, did not explode ontological categories.
In the Age of Wonder, mathematicians and
non-mathematicians alike looked past their astrolabes and into the heavens;
beyond the era's navigational achievements and into the dark forests of the New
World. Whatever Pascal may have wished, his adding machine could not compare
with the awe, astonishment, surprise, and fear—the wonder—that these
places evoked. Mathematicians focused on the well-established pleasures of
their craft, and it would be some time until their cabinet was filled with
curiosities that could capture the imagination in the same way as horned fish,
fluorescent birds, and the artifacts of entirely unsuspected parallel cultures.
3. Wonderful Mathematics.
Henle [19] has pointed out that
mathematics seems to pass through its own great Ages, corresponding in spirit
to great Ages in art and culture, but often with a considerable delay.
Descartes' influential belief that mathematics proceeds by its own internal
logic, on its own time, independent of a posteriori input, may help to
explain this apparent lag behind the Zeitgeist. Mathematics did
eventually experience a comparable Age of Wonder, but it was some 200 years
after Europe had been so transfixed by wunderkammern.
It was the 19th century when
mathematicians really began to wonder. The beginning of that century was
marked by the appearance on the mathematical horizon of the first ship from
what was undeniably a New World: Fourier's 1807 publication of Theory of the
Propagation of Heat in Solid Bodies [13, vol. 2]. The objects within its
hold, which Fourier called "functions" but which did not seem to be
anything of the sort, provoked an intense period of re-examination among
mathematicians. Fourier's functions seemed to be perfectly well-defined by a
method that was by then common practice—infinite series—and yet they exhibited
properties that could not be reconciled with the predominant mathematical
worldview. The series he used were trigonometric series, rather than the
familiar Taylor series. The functions they defined did not seem to be
determined by their derivatives, as Taylor series were. They were defined on
closed intervals, yet they behaved as if they were periodic. Their graphs did
not even look like functions—they jumped from one place to another without
passing through intermediate values. All of these properties were very
abnormal, and probably, it was thought, the result of some fundamental
misconception. The new objects were dismissed by Descartes' disciples, who felt
certain that this infidel mathematician and his ungodly "discoveries"
could be explained away. Excruciatingly, however, they could not. Despite the
best efforts of the Mathematical Inquisition, the terms of damnation could not
be agreed upon. Instead, mathematics worked itself into a very non-Cartesian
state of distraction. As the threads of Fourier's arguments were pulled apart,
they tangled around the feet of everything that was holy. Edicts and
proclamations were drawn up, but they could not dispel a profound anxiety that
was spreading throughout the whole of mathematical culture.
It is a credit to the great mathematicians
of the era that they, like their forebears from centuries before, responded to
the challenge with a sense of wonder. Lagrange, Abel, and Cauchy began to
dissect the new functions with a spirit reminiscent of the early anatomy
theaters. Before long, other explorers, map-makers, and curiosity collectors
would follow them on long voyages to a fertile new place called Analysis.
Younger mathematicians would come to their museums to ponder the skeletons of
strange new structures. They would wander through the curiosity cabinets of
Cauchy's Cours d'analyse [6, series 2, vol. 3]. When it was their time,
this next generation would follow curiosity's trade winds to an entirely New
Mathematical World.
Undoubtedly, the second half of the 19th
century was a time of tremendous mathematical treasures—wonderful
treasures. Cantor, returning from his solitary voyages, brought back the
varieties of infinity; Riemann sailed geometry to the higher dimensions where
it blends seamlessly with analysis; Weierstrass charted the ever-stranger
continuous but nowhere-differentiable functions, and Frege explored the deep
caverns of logic itself. What mathematics was, at this point, became a
thing of wonder in itself. The subject no longer seemed to proceed along
Descartes' ineluctable paths of reason, but rather through flights of the
imagination, inspired by dreams of what might be. Hans Hahn [17, p.
1956] later called this time a "crisis in intuition", and indeed it
was; but it was a crisis only for those who could not leave behind the solid
ground of Cartesian certainty. For those who were willing to test the wild
waters of the great new sea and give up their minds to the uncharted, it must
have been a wonderful, heady ride.
Consider, for example, the following
testimonial from Sylvester, delivered in an 1869 address [45, vol. 2, p. 654].
This was before many of the greatest explorations of the era, but already it
reflects a significant sea change away from Descartes and a strictly a
priori view of mathematics. The tone suggests the contentiousness of those
uncertain times.
|
We are told that "mathematics is
that study which knows nothing of observation..." I think no statement
could have been more opposite to the undoubted facts of the case; that
mathematical analysis is constantly invoking the aid of new principles, new
ideas and new methods, not capable of being defined by any form of words, but
springing direct from the inherent powers and activity of the human mind, and
from continually renewed introspection of that inner world of thought of
which the phenomena are as varied and require as close attention to discern
as those of the outer physical world, ...that it is unceasingly calling forth
the faculties of observation and comparison, that one of its principal
weapons is induction, that it has frequent recourse to experimental trial and
verification, and that it affords a boundless scope for the exercise of the
highest efforts of imagination and invention. ...Were it not unbecoming to
dilate on one's personal experience, I could tell a story of almost romantic
interest about my own latest researches in a field where Geometry, Algebra,
and the Theory of Numbers melt in a surprising manner into one another. |
As analysis began to mix inextricably with
geometry and the other branches of mathematics, the curiosities multiplied. New
results stretched the limits of imagination. Consequently, mathematicians began
to build intricate models out of wood, string, and plaster, transforming the
far horizons of their explorations into finite forms that others might
contemplate.
|
|
Figure 2. Clebsch's Diagonal Surface: Wonderful.
Fourier himself would have appreciated the
spirit in which these models were created, for he had always believed in the
power of mathematics to elucidate what could not be experienced directly. He
had written [14, p. 8]:
|
...if man wishes to know the aspect of the
heavens at successive epochs separated by a great number of centuries, if the
actions of gravity and of heat are exerted in the interior of the earth at
depths which will always be inaccessible, mathematical analysis can yet lay
hold of the laws of these phenomena. It makes them present and measurable,
and seems to be a faculty of the human mind destined to supplement the
shortness of life and the imperfection of the senses; and what is still more
remarkable, it follows the same course in the study of all phenomena; it
interprets them by the same language, as if to attest the unity and
simplicity of the plan of the universe, and to make still more evident that
unchangeable order which presides over all natural causes. |
The movement by mathematicians to build
intricate representations of exotic mathematical destinations was paralleled by
a wider 19th century educational movement to promote the use of much
simpler models in the universities and public schools. Some of the currents in
19th century mathematics education reform that led to this
development have been documented by Kidwell [26]. In the United States, for
example, sets of geometric solids were sold to the newly established common
schools in order to codify the impression of a common curriculum. The market
for these models was maintained well into the early parts of the 20th
century with fervent calls for, successively, "Object-Oriented
Instruction", "Technical Training", "Art Education",
and "Exact Thinking". The business eventually diversified into much
more lucrative catalogues of "Mathematical Apparatus", which included
everything from finely-crafted orreries and tellurians to the latest in elegant
"Pointing Rods".
There was money to be made in outfitting
mathematics departments with the instant educational cachet of a fine set of
models, but there was also a genuine belief in their inherent instructional
value, even among those who were doing the selling. This belief—that
mathematical models could help to develop essential intuition about difficult
analytic constructions—originated with two very influential mathematicians:
Gaspard Monge in France and Felix Klein in Germany. Together, they set the
standard for the way that mathematics was taught in Europe and America
throughout the 19th century. Monge is known as the father of
differential geometry, and his efforts in the early 1800s to classify surfaces
by the motions of lines, along with his "descriptive geometry" for
representing three-dimensional surfaces in two-dimensions, led naturally to the
construction of elaborate models made of tightly stretched strings. One of his
students, Théodore Olivier, built some of the most beautiful mathematical
models ever made. He also made some money in the process: the models were
expensive. Olivier sold them to the emerging technical schools in the United
States, which were attempting to emulate the example of Monge and the École
Polytechnic. Klein came along later in the century, promoting the use and
construction of mathematical models in graduate education, and the first
research universities in the United States did their best to follow the
European lead. Klein and his colleague Alexander Brill established a Laboratory
for the Construction of Mathematical Models in Munich, and the labors of their
graduate students were reproduced and sold world-wide by Brill's brother
Ludwig.
|
|
Figure 3. Klein's Munich Wunderkammern.
It was thus through a combination of
free-spirited mathematical exploration, educational idealism, and conspicuous
commercialism that the mathematical descendants of the wunderkammern
came into being. Schools proudly displayed their newly acquired models, to show
that they were up-to-date with the latest mathematical discoveries and
the most progressive educational trends. The models were often housed in
elaborately crafted cabinets, made of fine wood. The models themselves sat on
pedestals within the cabinets, sometimes on lush carpets of velvet. When they
were taken from their case and into the classroom, they were presented, by all
accounts, with great ceremony. Teachers and students making note of the
Cartesian principles that the models were meant to demonstrate couldn't help
but marvel at them—the treasures of the New World.
By the turn of the 20th century,
mathematical wunderkammern had proliferated across Europe and America.
Their spell, however, eventually lost its hold on the mathematical community.
Economic realities in the early part of the 20th century made the
acquisition of such "treasures" an increasingly difficult
proposition, and the market for finely-crafted models, as well as the
finely-crafted theories of education that went with them, fell off.
Entrepreneurs jumped in, and for a while cheaper knock-offs supplied the
diminishing demand. The increasingly clumsy constructions, however, could no
longer capture the collective imagination. Mathematicians had gotten down to
the hard work of sorting and classifying the accumulated discoveries of their
great Age of Exploration, and traditional Cartesian work ethics were once again
the mathematical vogue: skepticism, circumspection, and careful linear argument.
Educators also gathered themselves together, returning to an emphasis on
"fundamental skills". A purposefulness settled on the century, and
dust began to settle on the wunderkammern.
|
|
Figure 4. Johns Hopkins University, ca. 1895.
4. Whither Wonder?
The demise of the mathematical wunderkammern
was fated, inevitably, by larger historical forces that had been in motion for
centuries. The Enlightenment, with its emphasis on positivist inquiry and
empirical science, had come rushing through the 19th century and
into the 20th. Its great legacy, the Scientific Method, sought to
draw careful boundaries around what had once been wonderful, charting
intellectual continents of human dimension on a grid of comprehensible design.
The ability to map Nature became as much a source of wonder as Nature itself. Commerce
became Industry, and the success of the Scientific Method encouraged the belief
that everything inconvenient and unruly in Nature could eventually be
out-reasoned.
There were differences of opinion about
this, of course. Perhaps nothing symbolized the opposing world-view so much as
the Romantic movement in 19th century poetry, and perhaps no one
symbolized that movement so much as Samuel Coleridge. During a period absent of
muse, he wrote in his notebooks [22, p. 301]:
|
In my long illness I had compelled into
hours of Delight many a sleepless, painful hour of Darkness by chasing down
metaphysical Game—and since then I have continued the Hunt, till I found
myself unaware at the Root of Pure Mathematics—and up that tall smooth Tree,
whose few poor branches are all at its very summit, am I climbing by pure
adhesive strength of arms and thighs—still slipping down, still renewing my
ascent.—You would not know me!—all sounds of similitude keep at such a
distance from each other in my mind, that I have forgotten how to make
a rhyme—I look at the Mountains (that visible God Almighty that looks in at
all my windows) I look at the Mountains only for the Curves of their
outlines; the Stars, as I behold them, form themselves into Triangles—and my
hands are scarred with scratches from a Cat, whose back I was rubbing in the
Dark in order to see whether the sparks were refrangible by a Prism. The Poet
is dead in me—my imagination (or rather the Somewhat that had been
imaginative) lies, like a Cold Snuff on the circular Rim of a Brass
Candle-stick, without even a stink of Tallow to remind you that it was once
cloathed and mitred with Flame. That is past by!—I was once a Volume of Gold
Leaf, rising & riding on every breath of Fancy—but I have beaten myself
back into weight and density, & now I sink in quicksilver, yea, remain
squat & square on the earth amid the hurricane, that makes Oaks and
Straws join in one Dance, fifty yards high in the Element. |
There is, after all, something in the
triumph of Science that is fundamentally opposed to the essential requirements
of wonder. Wonder requires a diminished sense of oneself and one's
capabilities. The historian Adalgisa Lugli has noted [29, p. 123] that:
|
Wonder is a meta-historical category and
extends up to the end of the eighteenth century. It is defined primarily in
its didactic sense, as a form of learning—an intermediate, highly particular
state akin to a sort of suspension of the mind between ignorance and
enlightenment that marks the end of unknowing and the beginning of knowing. |
The great Age of mathematical wunderkammern
was only a back-eddy in the larger currents of 19th century Science that were
carrying the rest of the world over and around the "problems" in its
path. It is not surprising that mathematicians, true to Science and forever
beholden to manifestations of Cartesian progression, could not abide by their
disorderly collections of curiosities for very long. They sought to understand
the wonders, to put them in orderly mathematical contexts. After a century of
dissection and classification, the elaborately constructed models now
collecting dust in so many neglected cabinets look almost quaint, like the
mementos of a faded romance.
Has wonder, then, finally disappeared
beneath the flood of Science? The source of the flood, the rush toward
Enlightenment and its ideals, has been diverted many times during the past
century. The idea of Progress as Destiny has been battered about in political,
economic, and social turbulence. In uncertain currents, the belief that Science
would always find the true heading—implicit in Newton's mechanics, but not in
Heisenberg's—has been called into question. Finally, and irrevocably, the
twisted roots of Science were revealed in the brilliant light of the Atomic
Bomb. As a result, uncertainty and disillusionment have shaped the past century
as much as the ideals of the Enlightenment.
Wallace Stevens, the great philosophical
poet of this past century, laments the legacy of living in the grip of a Method
that is forever doomed by its inadequacies [44, p. 128]:
|
Oh! Blessed rage for order, pale Ramon, |
There is, however, something essentially new
in this kind of uncertainty. Whereas in past centuries there may have been more
general unknowing, this was accepted; it was not in conflict with previous
experience. Before the Enlightenment, there was not the expectation that
everything could be understood and controlled. Now, in the post-Enlightenment,
the loss of certainty feels like the result of some sort of guilty excess, and
there is an air of contrition surrounding every attempt to re-establish the Cartesian
course. Mathematics has not been exempt: Attempts to control perceived excesses
have consistently found their way into the debates on mathematics education. If
the past century has revealed a fundamentally chaotic dynamic at the interface
between Nature and the human mind, then (the arguments go, consciously or not)
it must be banished from mathematics, in much the same way that the
"misconceptions" of Galileo and Fourier were banished. The efforts
are as futile now as they were then.
Like Lagrange, Abel, and Cauchy before
them, there are mathematicians at the turn of this new millennium who recognize
the fundamental sea change that has taken place, and are willing to accept it
with wonder. While mathematics and science have undoubtedly helped to create
the late 20th century aesthetic of containment and control,
mathematicians and scientists also have a long history of fighting such
strictures, especially when they begin to look like hegemony. Einstein didn't like
the uncertainties of quantum mechanics, but he could still say that [8, p. 11]:
|
The most beautiful experience we can
have is the mysterious. It is the fundamental emotion that stands at the
cradle of true art and true science. Whoever does not know it can no longer
wonder, no longer marvel, is as good as dead. |
Richard Feynman noted [12, p. 248]:
|
It is our responsibility as scientists,
knowing the great progress which comes from a satisfactory philosophy of ignorance,
the great progress which is the fruit of freedom of thought, to proclaim the
value of this freedom; to teach how doubt is not to be feared but welcomed
and discussed; and to demand this freedom as our duty to all coming
generations. |
As long as there is "unknowing",
there will be a recognition of the need for wonder.
In modern mathematics and mathematics
education, the pervasive presence of "technological" curiosities is
once again calling into question the Cartesian ideal of rigorously linear exposition.
Computer investigations are inherently non-linear experiences, tossing the
mathematical explorer about in a seemingly limitless sea of information. Even
the most timid students of mathematics, however, can ably surf the Web. The
numerical and graphical processing powers of computers have taken
mathematicians to previously unimagined worlds, and allowed them to return with
fantastic new treasures. There will continue to be, as there has always been,
time to apply the lessons of history, and to sort, connect, and classify these
marvelous new objects. It would seem a shame, however, with such fine galleons
harbored on the desktops in almost every modern mathematics department, not to
go along on the voyages, open to the wonder of what may lie ahead.

Figure 5. The Modern Wunderkammer.
|
What's
in Your Wunderkammer?
Shown here are the models in the
mathematical wunderkammer located in the Department of Mathematics at
the University of Arizona. Like those in most modern mathematics departments,
the collection is a combination of locally-made student and faculty projects
together with a variety of commercially produced models. Sadly, a century
since their Golden Age, many of the models are in disrepair and much of their
documentation has been lost. However, some recent detective work, with the
help of the Smithsonian Institution in Washington, has helped the department
identify models by the American educators W. W. Ross and R. P. Baker in the
collection. Working in the early decades of the 20th century, both
are acknowledged as having produced models of generally higher quality than
the majority of those made in America at the time. Some of the examples in
the University of Arizona collection are apparently quite rare, and
discovering their history was an exciting adventure for many in the
department. The detective work continues, and the models and the cabinet have
recently undergone a complete refurbishing. You can view the models, and
follow the latest developments in their story, at: http://www.math.arizona.edu/~models What's in your wunderkammer? What strange specimens are collecting dust in some dark corridor of your department? Or have you already discovered some of the history of your local collection, and would like to share it with others? Send your photos, questions, and stories to wmueller@alum.mit.edu. |
References:
1. Fulton H. Anderson, Francis Bacon, His Career and Thought, USC
Press, Los Angeles, 1962.
2. R. P. Baker, Mathematical Models, Iowa City, 1931.
3. David Bressoud, A Radical Approach to Real Analysis, The
Mathematical Association of America, Washington, D.C., 1994.
4. Florian Cajori, Mathematics in Liberal Education, Christopher
Publishing, Boston, 1928.
5. Florian Cajori, The Teaching and History of Mathematics in the
United States, Bureau of Education, Government Printing Office, Washington
D. C., 1890.
6. Augustin Cauchy, Oeuvres complètes d'Augustin Cauchy,
Gauthier-Villars, Paris, 1882.
7. René Descartes, Oeuvres de Descartes, Charpentier, Paris,
1850.
8. Albert Einstein, Ideas and Opinions, Crown, New York, 1954.
9. Thomas Everard, Stereometry, Or, The Art of Gauging Made easie by
the Help of a New Sliding-Rule Which Shews The Area's of Circles in Gallons and
Barrelrs and the Square and Cube-Root of any Number under 100000 by inspection;
and also Resolves many other Arithmetical Problems without Pen or Compasses:
With An Appendix Of Conick Sections, In Which The Nature and Original of
Several Solids (frequently mentioned in Books of Gauging) is Explained, and
their Magnitudes compared, Peacock, London, 1689.
10. Howard Eves, An Introduction to the History of Mathematics,
Saunders College Publishing, Philadelphia, 1983.
11. J. Ewing, ed., A Century of Mathematics Through the Eyes of the
Monthly, The Mathematical Association of America, Washington, D.C., 1994.
12. Richard Feynman, The Value of Science, in What Do You Care What
Other People Think?, Bantam Books, New York, 1988.
13. Jean Baptiste Joseph Fourier, Oeuvres de Fourier,
Gauthier-Villars et fils, Paris, 1888.
14. Jean Baptiste Joseph Fourier, Analytical Theory of Heat,
Cambridge, 1878.
15. Galileo Galilei, Considerazioni al Tasso, in Scritti letterari,
Florence, 1933.
16. Stephen Greenblatt, Marvelous Possessions: The Wonder of the New
World, University of Chicago Press, Chicago, 1991.
17. Hans Hahn, The Crisis in Intuition, in The World of Mathematics,
James R. Newman, ed., Simon and Schuster, New York, 1956, pp.1956-1976.
18. William Hawney, Hawney's Complete Measurer, Or, The whole Art of
Measuring: Being A Plain And Comprehensive Treatise On Practical Geometry And
Mensuration: Preceded By Decimal And Duodecimal Arithmetic, And The Extraction
Of The Square And Cube Root: Adapted To The Use Of Schools And Persons
Concerned In Measuring, Gauging, Surveying, &c., Cochran and
McLaughlin, Philadelphia, 1801.
19. Jim Henle, Classical Mathematics, Amer. Math. Monthly 103
(1996) 18-29.
20. D. Hilbert and S. Cohn-Vossen, Geometry and the Imagination,
Chelsea Publishing Company, New York, 1952.
21. J. Holbrook, Apparatus Designed for Families, Schools, Lyceums and
Academies, Allen and Ticknor, Boston, 1833.
22. Richard Holmes, Coleridge: Early Visions, 1772-1804, Hodder
& Stoughton, London, 1989.
23. Oliver Impey and Arthur Macgregor, editors, The Origins of
Museums: The Cabinet of Curiosities in Sixteenth and Seventeenth Century Europe,
Clarendon Press, Oxford, 1985.
24. Phillip S. Jones, editor, A History of Mathematics Education in
the United States and Canada, National Council of Teachers of Mathematics,
Washington D. C., 1970.
25. Joy Kenseth, editor, The Age of the Marvelous, Hood Museum of
Art, Hanover, New Hampshire, 1991.
26. Peggy Kidwell, American Mathematics Viewed Objectively: The Case of
Geometric Models, in Vita Mathematica, Ronald Calinger, ed., The
Mathematical Association of America, Washington, D.C., 1996, pp. 197-207.
27. Morris Kline, Mathematical Thought from Ancient to Modern Times,
Oxford University Press, New York, 1972.
28. Sally Gregory Kohlstedt, Parlors, Primers, and Public Schooling:
Education for Science in Nineteenth Century America, Isis 81, 1990.
29. Adalgisa Lugli, Inquiry as Collection: The Athanasius Kircher Museum
in Rome, Res 12 (Autumn, 1986) 109-124.
30. Antonie M. Luyendijk-Elshout, Death Enlightened: A Study of Frederik
Ruysch, Journal of the American Medical Association 212, 1970.
31. N. Luzin, Function: Part I, The Amer. Math. Monthly 105, 1998,
pp.59-67.
32. Arthur Macgregor, editor, Tradescant's Rarities: Essays on the
Foundation of the Ashmolean Museum, 1683, with a Catalogue of the Surviving
Early Collections, Clarendon Press, Oxford, 1983.
33. Rushell L. McCarney, The Evolution of Mathematics at Hood College,
unpublished article, 1993.
34. William Mueller, Lost Geometry: An Introductory Essay, for the Catalogue
of Mathematical Models at the University of Arizona, Department of Mathematics,
University of Arizona , Tucson, 1998.
35. William Mueller, Reform Now, Before It's Too Late!, to appear in The
Amer. Math. Monthly 108, 2001.
36. Robert P. Multhauf, editor, A Catalogue of Instruments and Models
in the Possession of the American Philosophical Society, American
Philosophical Society, Philadelphia, 1961.
37. Karen Hunger Parshall and David E. Rowe, The Emergence of the
American Mathematical Research Community, 1876-1900: J. J. Sylvester, Felix
Klein, and E. H. Moore, The American Mathematical Society, Providence,
1994.
38. Karen V. H. Parshall and David E. Rowe, Embedded in the Culture:
Mathematics at the World's Columbian Exposition of 1893, The Mathematical
Intelligencer 15, 1993.
39. Blaise Pascal, Oeuvres complètes, Gallimard, Paris, 1954.
40. W. D. Reeve, editor, Multi-Sensory Aids in the Teaching of
Mathematics, Bureau of Publications, Teacher's College, Columbia
University, New York, 1945.
41. David L. Roberts, Albert Harry Wheeler (1873-1950): A Case Study in the
Stratification of American Mathematical Activity, Historia Mathematica
23, 1996.
42. David L. Roberts, Select Bibliography on Geometric Models,
Visualization, and the Teaching of Mathematics, unpublished bibliography,
1992.
43. W. W. Ross, Mensuration Taught Objectively with Lessons on Form,
Freemont, OH, 1891.
44. Wallace Stevens, The Idea of Order at Key West, in Collected Poems,
Vintage, New York, 1982.
45. James Joseph Sylvester, Presidential Address to the mathematical and
physical section of the British Association for the Advancement of Science,
Exeter, 1869.
46. Deborah Jean Warner, Commodities for the Classroom: Apparatus for
Science and Education in Ante-bellum America, Annals of Science 45,
1988.
47. Lawrence Weschler, Mr. Wilson's Cabinet of Wonder, Pantheon
Books, New York, 1995.
MathSoft, Inc., 101 Main St., Cambridge, MA 02142