Linear Functions
Algebraic Representation![]()
|
f(x) = a + bx |
Algebraically, linear functions are a two parameter family. The parameter a is called the function's y-intercept and the parameter b is called the slope. Together, they completely determine a linear function's input-output behavior.
Notice that f(0) = a : The y-intercept is the output when the input is 0.
When a = 0 the function simplifies to y = f(x) = bx, or a proportional relationship between y and x with b as the constant of proportionality.
If (x0, y0) and (x1, y1) are any two input-output pairs for the function, then
![]()
That is, the slope gives the average rate of change between any two points. Since this rate is the same regardless of the input-output pairs chosen, we see that linear functions have a constant rate of change.
Larger values of b mean larger rates of change for the function; smaller values mean smaller rates. Positive values of b give positive rates of change, or increasing functions; negative values give decreasing functions.
When b = 0 the function simplifies to y = f(x) = a, or a constant function with the same output for every input.
|
That is, increasing any input x by a constant interval Dx changes the output by a constant interval bDx . Said another way, if the input changes by Dx , then the output changes by the slope times Dx . This is the property of linear functions that is most easy to recognize in modeling situations. |
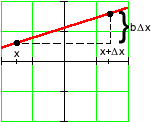
|
|
|
|
| Back to Contents | |